Answer to Problem 14
The answer is
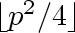 and the graph for which this bound is achieved is a
complete bipartite graph with partitions of size
and the graph for which this bound is achieved is a
complete bipartite graph with partitions of size
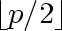 and
and
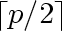 ,
respectively. Such a graph is shown here.
,
respectively. Such a graph is shown here.
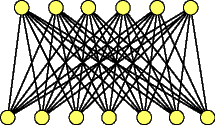
Figure 11:
This graph has no triangles (every path of length 2 is an induced subgraph) and among all such graphs with 13 vertices has the maximal possible number of edges: 42.
Now for the really hard part ... can you prove that this is true?
The proof